DMT Prime Factorization Revisited

In 2006, Marko A. Rodriguez came up with the idea of asking DMT entities to factor large numbers into primes to prove that the entities people experience while using DMT are real. In his paper, “A Methodology for Studying Various Interpretations of the N,N-dimethyltryptamine-Induced Alternate Reality” (a), he suggested using numbers with 7 ± 2 digits (which humans can easily remember).

In 2015, Scott Alexander wrote a great story using this idea as inspiration called “Universal Love, Said The Cactus Person” (a). The number Scott used for his story had 100 digits!

Gwern commented (a) on the piece with a Fermi estimate of how much it would cost to run this kind of prime factorization experiment. It came out to about $1k for running the study with an 800 digit number.

WHY DO THE NUMBERS KEEP INCREASING IN SIZE!?

Well, Scott probably chose his number for artistic effect, and the reason Gwern chose that large of a number is because…

“The smallest RSA number not yet factored is 220 digits (https://en.wikipedia.org/wiki/RSA_numbers#RSA-220). The RSA numbers themselves are useless for this experiment because if one did get the right factors, because it’s so extraordinarily unlikely for machine-elves to really be an independent reality, a positive result would only prove that someone had stolen the RSA answers or hacked a computer or something along the lines. RSA-768 was factored in 2009 using ~2000 CPU-years, so we need a number much larger; since Google has several million CPUs we might want something substantially larger, at least 800 digits.”

―Gwern

But after thinking about the experiment again with the author of the blog Applied Divinity Studies (a), I realized that Gwern’s demands for such a large number are only necessary if someone wants truly incontrovertible evidence to share with the rest of the world. If a trustworthy research group put out a study using numbers of the size Rodriguez suggested (6-8 digits), we should already be super impressed!

Why?

Because

I) there are sufficiently many 6-8 digit number with only two prime factors (>700,000), making it infeasible for memorization to give you an edge

II) factoring a 6-8 digit number with only two prime factors that aren’t sufficiently small (≥ 3 digits) is extremely difficult. The average person, let alone a mathematician, would not be able to solve this problem in 5-10 minutes in their head, and even with educated guessing, the odds of choosing the correct answer is < .08%

I explained)

There are 1,204 3-digit and 4-digit primes (confirm for yourself here (a)).

This means there are _1,204C₂ + 1,204 = 725,410 numbers with 6- to 8-digits that have exactly two prime factors that aren’t sufficiently small (≥ 3 digits).

I computed all those numbers and shuffled them at random. You can find a list of those numbers here: 6-8-digit-2-factor-numbers.txt.

If you want, you can choose any of those numbers, memorize it, and run the DMT prime factorization experiment.

You can check to see if you got the right answer afterward by running ‘factor X’ in WolframAlpha.

II explained)

What are the odds that you guess the correct answer (assuming you haven’t memorized a table of primes, but are sufficiently smart enough not to guess a number that is clearly composite (multiple of 2, 3, or 5)? In other words, how many 3-digit and 4-digits numbers are there that aren’t multiple of 2, 3, or 5?

There are 900 3-digit numbers and 9,000 4-digit numbers. 9,900 total.

• Half of those numbers (4,950) are even
• About 1/3 of those numbers (3,300) are a multiple of 3
• About 1/5 of those numbers (1,980) are a multiple of 5
• About 1/6 of those numbers (1,650) are a multiple of 2 and 3
• About 1/10 of those numbers (990) are a multiple of 2 and 5
• About 1/15 of those numbers (660) are a multiple of 3 and 5
• And about 1/30 of those numbers (330) are a multiple of 2, 3, and 5

Making sure not to double count anything, there are approximately 4,950 + 3,300 + 1,980 - 1,650 - 990 - 660 + 330 = 7,260 numbers that are a multiple of 2, 3, or 5.

So a reasonably smart person would be choosing from a set of 9,900 - 7,260 = 2,640 possible factors. One (if the 6-8 digit number is a perfect square) or two of those numbers would be in the correct factorization.

Meaning your odds of taking an educated guess at random and getting the correct answer is < 2 / 2,640 = .0758%.

The Actual Computational Advantages of DMT

If your main goal with this experiment is to confirm that extremely intelligent DMT entities from a hyperdimension or parallel universe are real, then asking for prime factors seems like a fine choice (so long as they’re willing to cooperate and reveal their intelligence!). And getting positive results from the experiment with any of the 6- to 8- digit numbers from the list I made is already very strong evidence (repeat the experiment a few times in a row for even stronger results) that something incredibly interesting is going on.

However, even with negative experimental results (either from DMT entities not being real or DMT entities being real but not willing to collaborate), we shouldn’t simply dismiss DMT consciousness as simply weird and call it a day.

Perhaps DMT consciousness does offer computational advantages for certain problems, but factoring large numbers is just not the right type of problem.

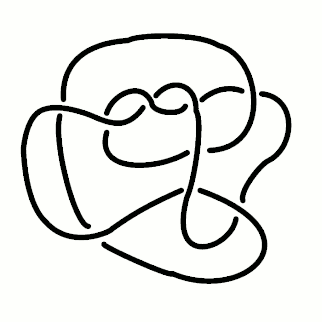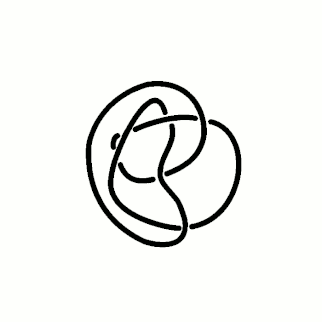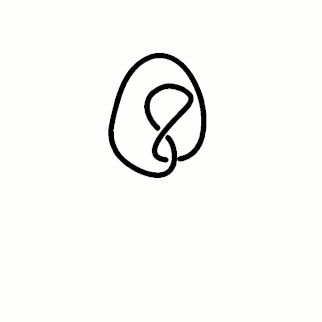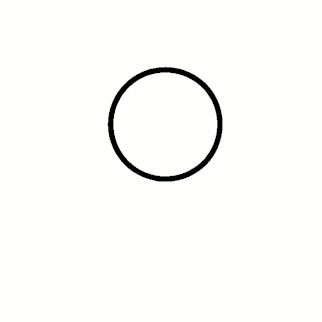
Maybe DMT consciousness helps you more easily tell whether some complex knot is equivariant with the unknot.

Unfolding a complex knot into the trivial knot, from Kuchtact (a)

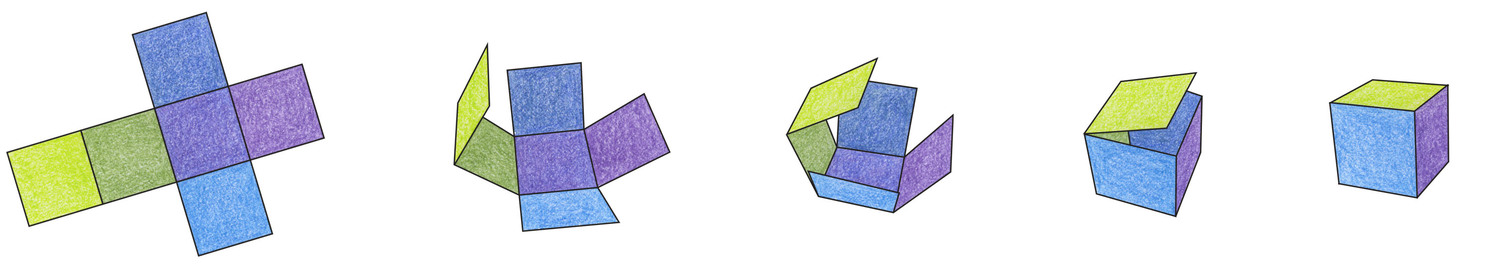
Or maybe DMT consciousness makes it easier to visualize how 2D plans will fold into 3D structures.

image from Stacy Speyer (a)

Or maybe at the end of the day, as Andrés Gómez Emilsson has described, DMT consciousness is just really freaking good at rendering hyperbolic geometry (a), perhaps with some universal love, and transcendent joy too.

Hard to say. More qualia research needed to determine the answer!